(click graph for a larger version)

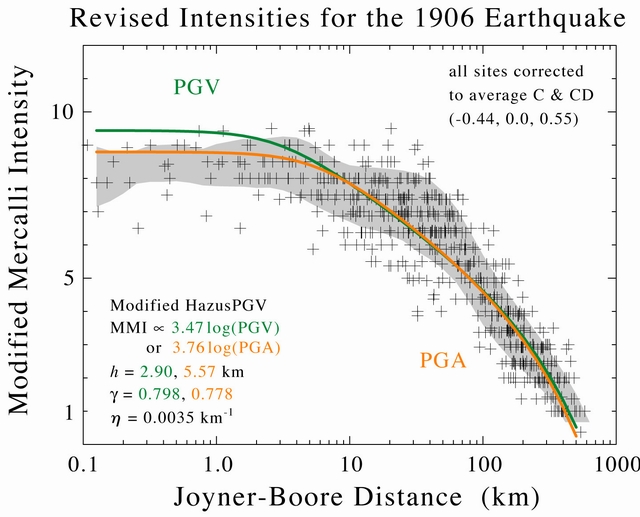
This plot shows the Modified Mercalli Intensities for ~ 600 sites plotted as a function of the Joyner-Boore distance from the fault trace (Joyner and Boore, 1979). The intensities are corrected for soil-type before plotting: the NERHP site class is determined from the Wills et al. (2001) geologic map of California. We have combined NEHRP-B and BC sites into a soft-rock class, NEHRP-C and CD sites into a soil class, and NEHRP-D and E sites into a soft-soil class. The intensity shifts for these aggregate soil/rock classes are obtained by averaging the residuals relative to C & CD sites. These shifts are written in the upper left hand corner of the plot: the soft-rock sites (B & BC) are deamplified by slightly less than half an intensity unit (-0.44) while the soft-soil sites (D & E) are amplified by slightly more than half an intensity unit (0.55).

The shaded area indicates the one standard deviation confidence interval for the running mean of the corrected intensity data. The intensities are effectively flat at distances near the fault (r_JB < 10 km), then falloff only slightly to r_JB > 80 km. There is some variability of the intensities at these intermediate distances. Beyond 100 km, however, the intensities behave very consistently, falling off sharply.

To generate the MMI ShakeMaps, we invert the relations between PGA (in %g) and PGV (in cm/s) and instrumental intensity derived by Wald et al. (1999)

I_mm = 3.66*log PGA + 1.99

I_mm = 3.51*log PGV + 2.35

to estimate PGA(I) and PGV(I) for each site and then follow the usual ShakeMap methodology. That is, we correct the peak motion to NEHRP-B, regress the data to estimate the source term (or bias), use this bias with the attenuation function to estimate peak ground motion at the false stations, interpolate a map of the peak motions for NEHRP-B, and finally apply the site amplifications derived from the geology.

The orange line shows the I_mm estimated as a function of Joyner-Boore distance using the Boore et al. (1997) relationship for PGA

lnPGA = - 0.313 + 0.527 (M-6) - 0.778 ln r_JB - 0.0035 r_JB - 0.371 ln(1396/605)

or

lnPGA = 0.946 - 0.778 ln r_JB - 0.0035 r_JB

which has been modified to fit the distances r_JB > 80 km by adding the exponential falloff exp[-0.0035 r_JB].

The green line shows the I_mm estimated as a function of Joyner-Boore distance using the Boore et al. (1997) relationship for PSA at 1 s,

lnPSA = - 1.133 + 1.036 (M-6) - 0.798 ln r_JB - 0.0035 r_JB - 0.698 ln(1406/605)

or

lnPSA = 1.320 - 0.798 ln r_JB - 0.0035 r_JB

which has also been modified to fit the distances r_JB > 80 km by adding the exponential falloff exp[-0.0035 r_JB]. Wald et al. (1999) use the 1982 Newmark-Hall relationship

PGV = 0.9467 PSA

to estimate PGV from PSA. The PGV curve overestimates the intensities at distances close to the fault. We note that ShakeMap uses PGV to estimate I_mm > 6, and PGA for I_mm ≤ 6.