U.S. GEOLOGICAL SURVEY
Scientific Investigations Report 2004–5237

Conceptual Model and Numerical Simulation of the Ground-Water-Flow System in the Unconsolidated Deposits of the Colville River Watershed, Stevens County, Washington

Back to Table of Contents

Model Limitations

A ground-water-flow model represents a complex, natural system with a set of mathematical equations that describe the system. Intrinsic to the model is the error and uncertainty associated with the approximations, assumptions, and simplifications that must be made. Hydrologic modeling errors typically are the consequence of a combination of (1) input data, (2) representation of the physical processes by the algorithms of the model, and (3) parameter estimation during the calibration procedure (Troutman, 1985). Examples of the three types of model errors and how those errors limit application of the model are as follows:

1. Data on types and thicknesses of hydrogeologic units, water levels, and hydraulic properties were taken from Kahle and others (2003) and represent only approximations of actual values. Most of the data were concentrated along the Colville River valley and in a few other populated areas, so that for most of the study area, little information is available to constrain the model. The hydraulic property data generally come from specific capacity tests, which typically measure drawdown at one time and at one pumping rate. Broad ranges of hydraulic property parameter values are possible. No short- or long-term data exist that provide information about how the system responds to changing, transient stresses such as increased ground-water pumping rates. With no long-term data, it is unknown if the simulated steady-state response is truly representative.
   
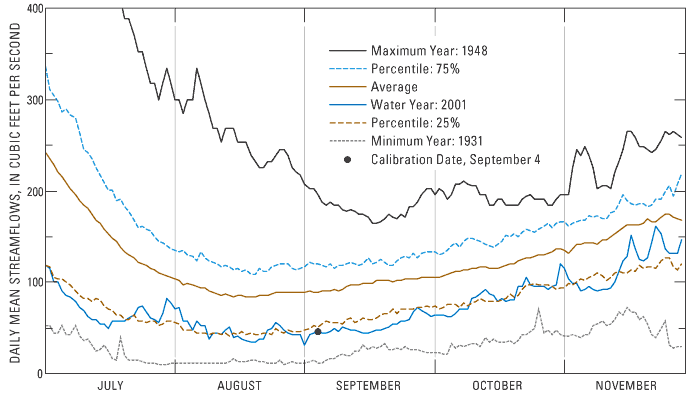
   Another possible deficiency is a lack of understanding of the geology at the mouth of the watershed. The complex geology near Kettle Falls could possibly control ground-water flow for a large part of the watershed. Much additional data would be required to understand the influence of alternative conceptual geologic conditions on the hydrologic conditions near Kettle Falls. Finally, there is a paucity of the data needed to explain the connection between the unconsolidated sediments and the bedrock. The conceptual model on which the numerical model is based presumes negligible or insignificant flow between the unconsolidated sediments and the bedrock and no flow from outside the Colville River Watershed. It is doubtful that the bedrock supplies a large source of water but an underestimation would result in model error.
   
   
2. A numerical model cannot represent completely, or "capture" all the physical processes of a watershed. Determining if a weakness in a simulation is attributable to input data error or model shortcomings is almost impossible, but the simplifying assumptions and generalizations that are incorporated into a model undoubtedly affect the results of the simulation. The assumption that the system was in steady state during September 2001, and the associated implications, clearly is important. The simulation period does not represent average conditions but rather the 25th percentile of low-flow periods (fig. 24). The system is considered to be in dynamic equilibrium, but flows in the Colville River at Kettle Falls in September 2001 ranged from 32 to 73 ft³/s. Between mid-August and November, flow increased by 73 ft³/s.
   
   If the regional, steady-state ground-water-flow model is used appropriately for the stated specific purposes, the effects of the simplifications can be limited. If the model is used for simulations beyond which it was designed, however, the generalizations and assumptions used could significantly affect the results.
   
   
3. Errors in parameter estimates occur when improper values are chosen during the calibration process. Various combinations of parameter values can result in low residual error, yet improperly represent the actual system. An acceptable degree of agreement between simulated and measured values does not guarantee that the estimated model parameter values reasonably represent the actual parameter values. The use of nonlinear regression and associated statistics, such as composite scaled sensitivities and correlation coefficients, removes some of the effects of non-uniqueness, but certainly does not eliminate the problem entirely.

Figure 24. Statistical summary of mean daily discharges at the Colville River at Kettle Falls, Washington (station 12409000) during July – November for 1923-2001 and for 2001.

Back to Table of Contents

For more information about USGS activities in Washington, visit the USGS Washington District home page .